Physical Address
304 North Cardinal St.
Dorchester Center, MA 02124
Physical Address
304 North Cardinal St.
Dorchester Center, MA 02124
Fractals, complex mathematical curves that exhibit the same shape at all levels of size, have captured the attention of scientists and artists alike. These intricate patterns, characterized by their self-similarity and infinite complexity, have become a subject of fascination and exploration. From the microscopic to the gigantic, fractals provide a unique lens through which we can understand and describe the jagged lines and rough surfaces that exist in our world.
One of the most captivating aspects of fractals is their prevalence in the natural world. Consider the leafy fronds of a fern or the geometrically patterned buds on Romanesco broccoli. Upon closer inspection, we discover that these structures consist of repeating smaller versions of themselves, forming intricate fractal shapes. This repeating pattern, observed at different scales, showcases the self-similarity that defines fractals.
Moreover, the mesmerizing beauty of snowflakes can be attributed to their fractal nature. As snowflakes form, they follow fractal patterns, resulting in their unique and intricate designs. Each snowflake is a testament to the infinite complexity and delicate balance found in nature.
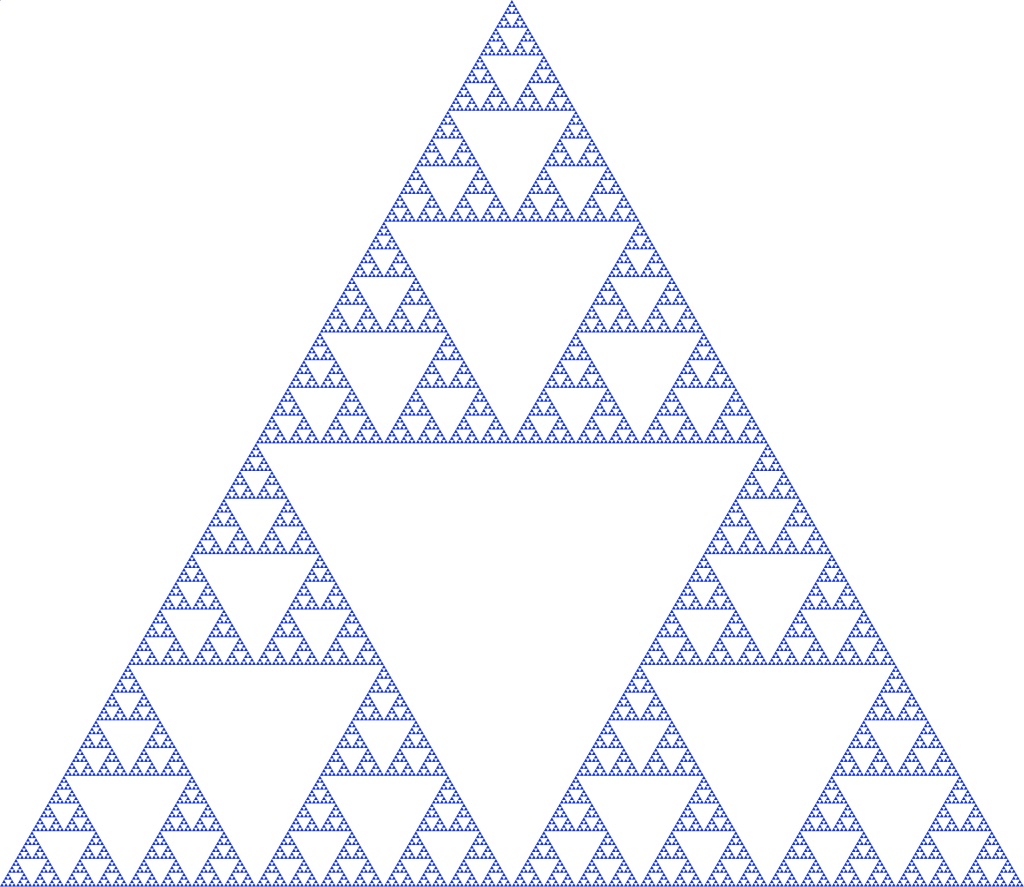
To delve deeper into the world of fractals, we can turn our attention to the Sierpinski triangle. This simple yet captivating fractal is formed by dividing an equilateral triangle into four smaller equilateral triangles, which are then further subdivided into smaller ones. The process continues indefinitely, creating a mesmerizing pattern that repeats at smaller and smaller scales.
By engaging in the act of drawing the Sierpinski triangle, we gain a visual understanding of how fractals can be constructed. As we meticulously fill in the shape with smaller versions of itself, we witness the power of repetition and self-similarity in creating intricate geometric patterns.
While our ability to draw fractals by hand is limited, computers have revolutionized our understanding and visualization of these complex shapes. With the aid of computational power, we can explore fractals in greater detail and witness their infinite complexity unfold before our eyes.
The Mandelbrot set, a well-known example of a fractal pattern, showcases the intricate and ever-changing nature of these geometric shapes. Through the use of algorithms and mathematical calculations, computers can generate stunning visual representations of the Mandelbrot set, revealing its morphing, twisting, and spiraling patterns.
The allure of fractals extends beyond the realm of mathematics and science. Artists have embraced these geometric patterns, incorporating them into their works to create visually captivating pieces. The intricate fractal designs have also found their way into computer-generated special effects in movies, adding an otherworldly and mesmerizing atmosphere to the big screen.
Furthermore, the study of fractals has provided valuable insights in various scientific fields. Fractal geometry has been applied to describe natural phenomena such as coastlines, mountain ranges, and even the human circulatory system. The self-similarity and scaling properties of fractals have proven to be powerful tools in understanding the complexity and interconnectedness of the world around us.
As we delve deeper into the world of fractals, we uncover a universe of infinite complexity and beauty. From the smallest snowflake to the grandest landscapes, fractals shape our understanding of the natural world and provide a glimpse into the underlying mathematical principles that govern our reality. Through the exploration of fractals, we unlock a deeper appreciation for the intricate patterns that surround us and gain a new perspective on the interconnectedness of all things.
The exploration of fractals and their intricate patterns has had a profound impact on various aspects of our world. From art and design to scientific research, the understanding and application of fractals have opened up new avenues of creativity and knowledge. Let us delve into the effects that the study of fractals has had on our society.
The discovery of fractals has provided artists with a new source of inspiration and a unique way to express their creativity. The intricate and mesmerizing patterns found in fractals have been incorporated into various art forms, including paintings, sculptures, and digital art. Artists have embraced the self-similarity and infinite complexity of fractals, creating visually captivating pieces that captivate the imagination and evoke a sense of wonder.
Fractal art has not only enriched the artistic landscape but has also found its way into popular culture. The mesmerizing and otherworldly atmosphere created by fractal patterns has been utilized in movies, video games, and visual effects, transporting audiences to fantastical realms and immersing them in visually stunning experiences.
The study of fractals has revolutionized our understanding of complex systems in various scientific disciplines. Fractal geometry has been applied to describe and analyze natural phenomena, such as the branching patterns of trees, the formation of coastlines, and the intricate network of blood vessels in the human body.
By utilizing fractal analysis, scientists have gained insights into the underlying patterns and processes that govern these natural systems. Fractals have provided a powerful framework for understanding the scaling properties, self-similarity, and intricate dynamics of complex systems, leading to advancements in fields such as physics, biology, and environmental science.
The understanding of fractals has also played a crucial role in technological advancements. Fractal-based algorithms and models have been employed in various fields, including computer graphics, data compression, and signal processing.
Computer-generated imagery (CGI) in movies and video games has greatly benefited from the application of fractals. The intricate and ever-changing patterns of fractals have been used to create realistic landscapes, textures, and special effects, enhancing the visual experience for audiences worldwide.
Furthermore, fractal-based compression algorithms have revolutionized the storage and transmission of digital data. By exploiting the self-similarity and redundancy within complex datasets, these algorithms enable efficient compression and decompression, reducing file sizes without significant loss of information.
The study of fractals has deepened our understanding of the natural world and our place within it. By recognizing the presence of fractal patterns in natural phenomena, we gain a greater appreciation for the interconnectedness and complexity of our environment.
Fractals serve as a reminder that the same fundamental principles and patterns can be found at different scales, from the microscopic to the macroscopic. This realization fosters a sense of awe and reverence for the beauty and intricacy of nature, encouraging us to protect and preserve our planet.
The exploration of fractals has unveiled a world of infinite complexity and beauty that exists within our reach. From inspiring artistic creations to advancing scientific knowledge and technological innovations, fractals have left an indelible mark on our society.
As we continue to unravel the mysteries of fractals, we gain a deeper understanding of the patterns and processes that shape our world. The study of fractals serves as a testament to the power of mathematics and its ability to unlock the secrets of nature, inspiring us to explore, create, and appreciate the infinite wonders that surround us.
If you’re wondering where the article came from!
#